Kerr black hole
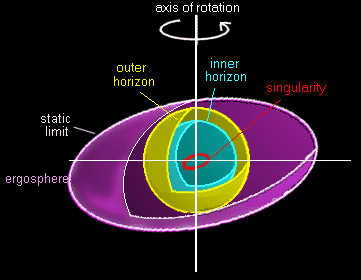
Figure 1. Main features of a Kerr black hole. Credit: N. Rumiano.
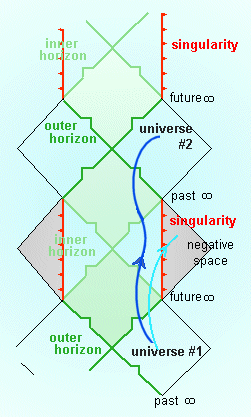
Figure 2. Penrose diagram of a Kerr black hole. Credit: N. Rumiano
A Kerr black hole is a type of black hole that possesses only mass and angular momentum (but not electrical charge – the third possible property of a black hole). In other words, a Kerr black hole is an uncharged black hole that rotates about a central axis. It is named after the New Zealand mathematician Roy Kerr who, in 1963, became the first person to solve the field equations of Einstein's general theory of relativity for a situation of this kind.
Kerr black holes are probably the commonest in nature, since the massive stars from which they typically form possess rotation (but no overall charge) before they collapse at the end of their lives. By the principle of conservation of angular momentum, much of this spin is then retained by the black hole following the star's terminal collapse.
A Kerr black hole has the following distinct regions (Figure 1):
Ergosphere
The ergosphere is an ellipsoidal region around a Kerr black hole in which the hole's rotation drags the spacetime continuum round with it – a phenomenon known as the Lense-Thiring effect or frame dragging – so that frames of reference are not stationary with respect to the rest of the universe. The ergosphere is bounded on the outside by the static or stationary surface, and on the inside by the outer event horizon.
If an object passes from normal space into the ergosphere it can still be ejected from the black hole by gaining energy from the hole's rotation. However, if an object crosses the event horizon, it will be sucked into the black hole and never escape.
Inside a Kerr black hole
At each event horizon the roles of space and time are reversed; so, in the case of a Kerr black hole space and time swap places twice. The singularity is ring-shaped and, except if approached on its equatorial plane, is repulsive. This fact is simply the result of the equations of Kerr's metrical geometry. The singularity is also a temporal one, so that it can be avoided. In theory, it is possible to escape from a black hole, although not by the same way you went in. On leaving the black hole you would find yourself either in a region of "negative space," the physical meaning of which is unclear, or in an entirely different universe.
The Penrose diagram in Figure 2, which shows the internal structure of a Kerr black hole, makes this clear. The dark blue path is of a traveler coming from the past in universe #1, crossing the outer horizon then the inner one, avoiding the singularity, crossing the horizons again, and finally emerging in another universe. The light blue path is of a traveler who crosses the singularity and thereby arrives in a "negative universe".
Warning to travelers!
Everything that has been said here is purely theoretical. The Kerr solution is very unstable, corresponding as it does to a black hole in complete isolation. The addition of extraneous matter, such as even the approach of a would-be traveler, could be enough to destabilize the Kerr solution and make travel through the black hole unrealistic. To properly investigate the feasibility of journeys past or through the singularities of black holes we need to be able to take quantum effects into account. However, this will require a quantum theory of gravity – one of the chief goals of contemporary theoretical physics.Compare with Schwarzschild black hole and Reissner-Nordstrom black hole.


