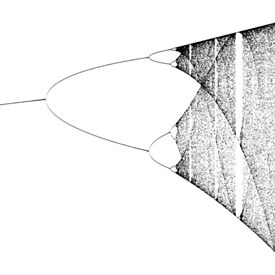
A bifurcation is the value of a smoothly varying control parameter, or the point in parameter space, at which the behavior of a dynamical system undergoes a qualitative change. For example, a simple equilibrium, or a fixed-point attractor, might give way to a periodic oscillation as the stress on a system increases. Similarly, a periodic attractor might become unstable and be replaced by a chaotic attractor.

To give a real-world example, from a dripping faucet at low pressure, drops fall individually at equal intervals. As the pressure is increased, however, the pattern of dripping abruptly changes so that two drops fall close together, with a longer interval before the next pair fall. In this case, a simple periodic process has given way to a periodic process with twice the period, a process described as "period doubling." If the flow rate of water through the faucet is increased still further, beyond the bifurcation point, often an irregular dripping is found and the behavior can become chaotic.