fraction
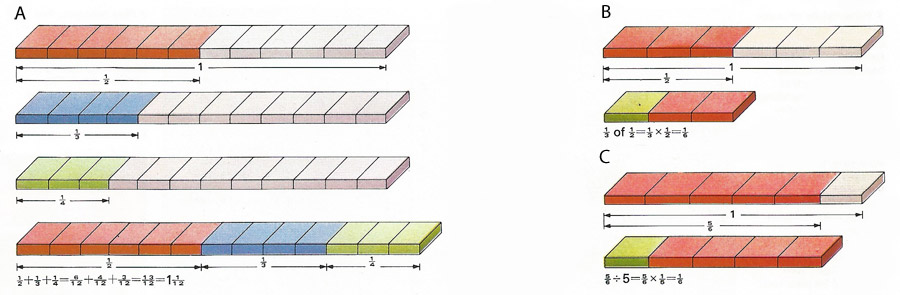
[A] Before fractions can be added they must all be expressed in terms of the same denominator. To add 1/2, 1/3, and 1/4 they must all be stated in twelfths (in this example, 12 is the lowest common denominator) as 6/12, 4/12, and 3/12. They can then be added to give 13/12, an improper fraction that simplifies to 1 1/12. This sum explains why it is impossible to divide anything into shares of 1/2, 1/3, and 1/4 – their sum is larger than 1.
[B] To multiply fractions merely multiply the numerators and then multiply the denominators. A third of a half is 1/3 × 1/2 = 1/6 (the same as a half of a third – order doesn't matter).
[C] To divide fractions invert the divisor and multiply. For example, 5/6 divided by 5 is 5/6 × 1/5 = 1/6, which is exactly the same as the quantity described as a third of a half, as shown in illustration B.
A fraction is a rational number that represents a part, or several equal parts, of a whole; examples include one-half, two-thirds, and three-fifths. The word comes from the Latin frangere, meaning "to break." A simple, common, or vulgar fraction is of the form a/b, where a (the numerator) may be any integer and b (the denominator) may be any integer greater than 0. If a < b, the fraction is said to be proper ("bottom heavy"); otherwise it is improper ("top heavy"). A solidus is the slanted line in a fraction dividing the numerator from the denominator. A decimal fraction has a denominator of 10, 100, 1000, etc.
In an algebraic fraction, the denominator, or the numerator and denominator, are algebraic expressions, for example x/(x 2 + 2). In a composite fraction, both the numerator and denominator are themselves fractions.
Two fractions a/b and c/d can be added, subtracted, multiplied, and divided according to the rules:
a/b + c/d = (ad + bc)/b/d
a/b – c/d = (ad – bc)/bd
a/b × c/d = ac/bd
a/b ÷ c/d = ad/bc
Egyptian fraction
An Egyptian fraction is a unit fraction, in other words, a fraction in which the numerator (the number on top) is 1. This type of fraction was the only kind used by the ancient Egyptians and appears extensively in the Rhind papyrus. Other fractions can be obtained by adding Egyptian fractions together; for example, 5/7 = 1/2 + 1/6 + 1/21. In 1201 Fibonacci proved that every rational number can be written as a sum of Egyptian fractions.


