Internet Encyclopedia of Science
GEOMETRY
A  B  C  D  E  F  G  H  I  J  K  L  M  N  O  P  Q  R  S  T  U  V  W  X  Y  Z

                   • HOME
• ABOUT
• CATEGORIES
• COPYRIGHT
• NEWSLETTER

  



line


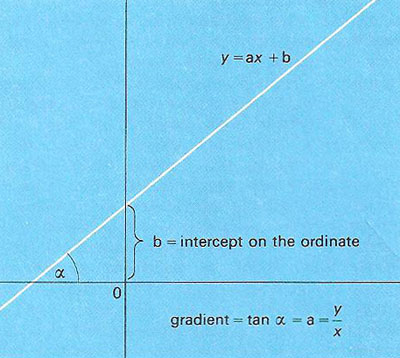
straight line graph
The graph of y = ax + b, the prototypical linear relationship
The shortest distance between any two points in Euclidean space. A line is implicitly a straight line. It has only one dimension, length, may be infinite in extent, and is the shortest distance between any two points.

Although in common parlance a straight line is not curved, it is a curve in the mathematical sense (i.e., a mapping of an infinite set of points into a space). Mathematically, a line may be determined by the presence of any two points in an n-dimensional space (where n is two or more). A line segment is a piece of a line with definite endpoints.

In two dimensional Cartesian coordinates, the equation of a line has the form y = ax + b, where a and b are constants.


Related category

   • GEOMETRY


Also on this site:

Encyclopedia of Alternative Energy & Sustainable Living
Encyclopedia of History


BACK TO TOP