parallelogram
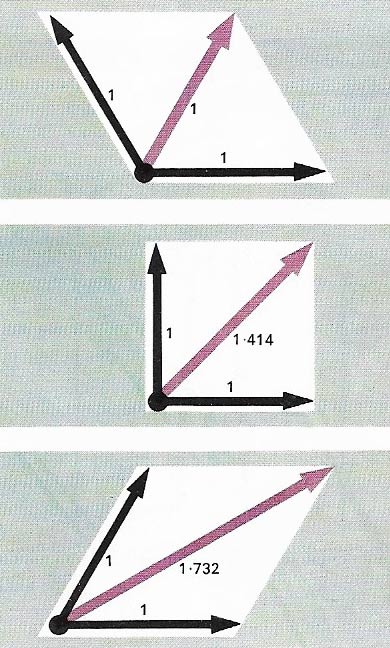
Parallelogram of forces. Two forces act simultaneously on an object in different directions. The resultant force, and the direction of any subsequent movement, is defined by the diagonal of a parallelogram whose sides are drawn parallel to the applied forces with lengths proportional to their magnitudes. This is an application of vector diagrams.
A parallelogram is a quadrilateral (four-sided figure) whose opposite sides are parallel, and whose opposite angles, therefore, are equal. The diagonals of a parallelogram bisect each other. A parallelogram of base b and height h has an area:
A = bh = ab sinA = ab sinB.
The height of a parallelogram is h = a sinA = a sinB. The sides a, b, c, and d and diagonals p and q of a parallelogram satisfy the equality:
p2 + q2 = a2 + b2 + c2 + d2
Special cases of a parallelogram are a rhombus, which has sides of equal length, a rectangle, which has two sets of parallel sides that are perpendicular to each other, and a square, which meets the conditions of both a rectangle and a rhombus.
parallelogram of forces
If a particle is under the action of two forces, which are represented in magnitude and direction by the two sides of a parallelogram drawn from a point, the resultant of the two forces is represented by the diagonal of the parallelogram drawn from that point.
parallelogram of velocities
If a body has two component velocities, represented in magnitude and direction by two adjacent sides of a parallelogram drawn from a point, the resultant velocity of the body is represented by the diagonal of the parallelogram drawn from that point. The parallelogram of velocities, like the parallelogram of forces, is a particular case of the parallelogram of vectors.
A familiar application of it is to find the resultant velocity of an object, such as a boat, that is subject to a current acting in a different direction in the one in which it is trying to move.
